VAN BILSEN, Arthur (2007): Evolutionary Games and Architecture
VAN BILSEN, Arthur (2007): Evolutionary Games and Architecture, En SPACEFIGHTER: The Evolutionary City Game, Winy Maas et al.
72
Thomas Schelling’s book The Strategy of Conflict (1960) launched his vision of game theory as a unifying framework for the social sciences (Nobel Prize Committee, 2005). Both topics, the analysis of human interaction and a unifying framework for the social sciences, are relevant to architectural and planning discourse.
72-73
Adaptationism is the paradigm that views organisms as complex adaptive machines whose parts have adaptive functions subsidiary to the fitness-promoting function of the whole.
There can be no doubt that game-theoretic analyses work in evolutionary theory. Why for instance, are the Redwood trees so tall? Each tree is looking out for itself, and trying to get as much sunlight as possible. If only they could get together and stop competing with each other for sunlight. But they cannot. Defection from such cooperative agreement is bound to pay off. (Dennett, 1995, p.254)
it is possible for simple rules to yield innovative results. Surprisingly, it will be challenging to interpret patterns evolving from simple rules. Especially on the global level of a pixelized earth one may find innovative and interesting patterns, assuming one has the mindset to interpret them. The mindset advocated here incorporates the fundamentals behind games, cellular automata, Universal Turing Machines, evolutionarily stable strategies, complexity, and how they are connected. It is in real-world multi-scaled and complex processes that contemporary architects and urban planners have to intervene. The evolutionary gametheoretic point of view will prove a valuable contribution to the glasses with which architects and planners can view the world.
73
Introduction
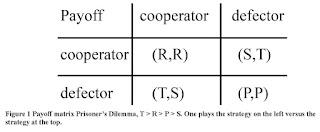
Games are situations involving parties with conflicting interests. A player (or agent) is, by definition, an entity with preferences. The utility function (Von Neumann and Morgenstern, 1947) denotes a measure of subjective psychological fulfilment, and assigns a score to each strategy. Players act so as to maximize their utility, which is better known as payoff. This can readily be shown through the payoff matrix, which is one of two principal ways to depict strategies’ payoffs (Fig 1 and 2). Playing for example strategy defect against cooperate yields payoff T for the defector and S for the cooperator (T>S).
74
The role that games may play is at least as a concept generating increased insight applicable in the analysis phase of a project. Considering the agents that make up a complex adaptive system as parties with conflicting interests, maximizing their individual fitness, is a useful language to understand the system’s degree of complexity. The conclusion could be drawn that the system is not as complicated as it seemed, simplifying the later design phases.
A relatively uncharted territory is found when considering many games, having many interactions. Prigogine proposes a useful classification. Systems with a small number of variables (or elements or games) which depend heavily on one another are classified as complex systems of order 1. Example: double pendulum. Systems with a large number of variables, which depend loosely or locally on one another are classified as complex systems of order 2. Example: traffic systems. Systems that have a large number of variables and a large dependence among them, are classified as complex systems of order 3. Example: market systems. All are sensitive to initial conditions. The conclusion is that most systems that are touched by urban planning and architecture are typically lower than order 3, and are not as problematic as one may think. For instance, an increasingly accurate model for traffic is evolving in recent years (Helbing and Nagel, 2004). Considering for instance four games with heavy interactions would constitute a complex system of order 1. A couple of thousand inter-dependent games, however, could already be classified as order 3.
The same classification can also be applied to the actor-field in which designs are created, changed and selected. If there are many actors having many interactions, everyone gets a say, and little will materialize, since the situation is too complex to solve or even unsolvable. If there are too little actors, their criterions do not sufficiently represent the much larger group of future users of the design. A balance is to be sought between stifling order and destructive chaos. Game theory can be applied at this interface to point out the strategy and maximize every actor’s payoff, or in other words: subjective psychological fulfilment. What is the “best” strategy in a given situation? In evolutionary game theory the concept of an evolutionarily stable strategy was introduced by John Maynard Smith to answer just that question.
75
Evolutionary Spatial Game Theory
An evolutionarily stable strategy (ESS) is a strategy that does well against copies of itself. The rationale for this is as follows. A successful strategy is one that dominates the population. Therefore it will tend to encounter copies of itself. Therefore it won’t stay successful unless it does well against copies of itself (Dawkins, 1989, p.282). In an architectural and planning context the planning or design strategy is played out between the plan or design and its users. In their environment people see themselves reflected, or want to see themselves reflected. This is sometimes called reflexivity. People and agents play games with themselves, as well as with their environments, including other people. While playing games, people who adopt an ESS, increase their chances on evolutionary fitness. In this setting urban planners and architects can be seen as players, increasing their fitness by designing an environment for users, who in turn try to increase their fitness using that environment. In the preceding, one may replace people with agents (Minsky, 1988, p.17), generalizing the argument even further to include mindless processes, involving replicator molecules, viruses and cellular automata (Wolfram, 1983). The latter is especially appealing for incorporating the spatial dimension, which is essential to architecture and urban planning and design. Nowak and May (1992) were the first to play the Prisoners Dilemma game on the spatial grid of a cellular automaton. See Fig. 3.
77-78
Each cell (or pixel) with a particular strategy (indicated by the cell’s colour) plays a game against all its eight neighbours, accumulating payoff. This of course holds for the neighbours as well. When all payoffs are calculated, squares of nine cells are considered. The strategy of the cell with the highest payoff, will become the strategy of the middle cell in the next step. This process continues indefinitely yielding many beautiful patterns such as in Fig. 3.
The unit of a cellular automaton (or game) is the cell, elsewhere in this book identified with a pixel, which can be in a fixed number of states. When in two states the states are referred to as ON and OFF, usually coloured black and white and interpreted as cells being alive and dead. In an urban planning context the states could be houses (RED), parks (GREEN) and infrastructure (BLUE). On this small scale the rules one imposes govern the allowed state changes over one time step. A game in an urban setting would for example show changing patterns of red, green and blue. The power of these simple automatons are not to be underestimated, as Conway has shown that with the Game of Life rule set, one can construct a Universal Turing Machine. A Universal Turing Machine can mimic any computable function. With such functions one could construct an intelligent chess program which plays itself. All of this can follow from simple rules governing cells in state ON or OFF.
78
Which rules between cells apply in an architectural and planning context? The rules synthesising a design can sometimes literally represent processes. At other times they can merely be efficient algorithmic vessels to achieve real processes. The choice of rules also influences the predictability of the system. Take for example one dimensional cellular automaton rule 146. It is not predictable if one analyses the fine structure, but surprisingly it is predictable when one looks on a coarser level (Isreali and Goldenfeld, 2004), despite its complexity! Other rules can easily be derived from strict boundary conditions with regard to regulation, law, safety, collective functions, health, such as a rule prohibiting housing near large industrial facilities and heavy traffic. The search space that these rules allow accessibility to is still vast. One may research the remaining search space with help from a computer in general or by playing a game.
Since we are talking about games that are evolutionary, they exhibit all four phases of the evolutionary algorithm: mutation, variation, growth, selection. We would make a critical error to see this as merely change, in which no real innovation can occur. After all, are we humans not also changing patterns of atoms? The rules of the game are like the laws of physics. And the live cells are like the most elementary particles known to physics today: quarks and leptons. On this premise one can compare the evolutionary game grid with physical reality.
Concluding remarks
Do urban planners and architects need this new pair of glasses? The conclusion I draw is far from complete. But I have shown that it is possible for simple rules to yield innovative results. It may be hard to interpret the patterns from the game-cell level (= pixel level), as it would be equally hard to interpret a human being from the atomic level! Therefore the global level of a pixelized earth may already yield some innovative and interesting patterns, only observable when one has the mindset and tools to interpret them. In any case games and cellular automata help understand the levels of scale involved, and the way they are connected. It is in real-world multi-scaled and complex processes that contemporary architects and urban planners have to intervene. The gametheoretic point of view is a valuable contribution to a new pair of glasses.
72
Thomas Schelling’s book The Strategy of Conflict (1960) launched his vision of game theory as a unifying framework for the social sciences (Nobel Prize Committee, 2005). Both topics, the analysis of human interaction and a unifying framework for the social sciences, are relevant to architectural and planning discourse.
72-73
Adaptationism is the paradigm that views organisms as complex adaptive machines whose parts have adaptive functions subsidiary to the fitness-promoting function of the whole.
There can be no doubt that game-theoretic analyses work in evolutionary theory. Why for instance, are the Redwood trees so tall? Each tree is looking out for itself, and trying to get as much sunlight as possible. If only they could get together and stop competing with each other for sunlight. But they cannot. Defection from such cooperative agreement is bound to pay off. (Dennett, 1995, p.254)
it is possible for simple rules to yield innovative results. Surprisingly, it will be challenging to interpret patterns evolving from simple rules. Especially on the global level of a pixelized earth one may find innovative and interesting patterns, assuming one has the mindset to interpret them. The mindset advocated here incorporates the fundamentals behind games, cellular automata, Universal Turing Machines, evolutionarily stable strategies, complexity, and how they are connected. It is in real-world multi-scaled and complex processes that contemporary architects and urban planners have to intervene. The evolutionary gametheoretic point of view will prove a valuable contribution to the glasses with which architects and planners can view the world.
73
Introduction
Games are situations involving parties with conflicting interests. A player (or agent) is, by definition, an entity with preferences. The utility function (Von Neumann and Morgenstern, 1947) denotes a measure of subjective psychological fulfilment, and assigns a score to each strategy. Players act so as to maximize their utility, which is better known as payoff. This can readily be shown through the payoff matrix, which is one of two principal ways to depict strategies’ payoffs (Fig 1 and 2). Playing for example strategy defect against cooperate yields payoff T for the defector and S for the cooperator (T>S).
74
The role that games may play is at least as a concept generating increased insight applicable in the analysis phase of a project. Considering the agents that make up a complex adaptive system as parties with conflicting interests, maximizing their individual fitness, is a useful language to understand the system’s degree of complexity. The conclusion could be drawn that the system is not as complicated as it seemed, simplifying the later design phases.
A relatively uncharted territory is found when considering many games, having many interactions. Prigogine proposes a useful classification. Systems with a small number of variables (or elements or games) which depend heavily on one another are classified as complex systems of order 1. Example: double pendulum. Systems with a large number of variables, which depend loosely or locally on one another are classified as complex systems of order 2. Example: traffic systems. Systems that have a large number of variables and a large dependence among them, are classified as complex systems of order 3. Example: market systems. All are sensitive to initial conditions. The conclusion is that most systems that are touched by urban planning and architecture are typically lower than order 3, and are not as problematic as one may think. For instance, an increasingly accurate model for traffic is evolving in recent years (Helbing and Nagel, 2004). Considering for instance four games with heavy interactions would constitute a complex system of order 1. A couple of thousand inter-dependent games, however, could already be classified as order 3.
The same classification can also be applied to the actor-field in which designs are created, changed and selected. If there are many actors having many interactions, everyone gets a say, and little will materialize, since the situation is too complex to solve or even unsolvable. If there are too little actors, their criterions do not sufficiently represent the much larger group of future users of the design. A balance is to be sought between stifling order and destructive chaos. Game theory can be applied at this interface to point out the strategy and maximize every actor’s payoff, or in other words: subjective psychological fulfilment. What is the “best” strategy in a given situation? In evolutionary game theory the concept of an evolutionarily stable strategy was introduced by John Maynard Smith to answer just that question.
75
Evolutionary Spatial Game Theory
An evolutionarily stable strategy (ESS) is a strategy that does well against copies of itself. The rationale for this is as follows. A successful strategy is one that dominates the population. Therefore it will tend to encounter copies of itself. Therefore it won’t stay successful unless it does well against copies of itself (Dawkins, 1989, p.282). In an architectural and planning context the planning or design strategy is played out between the plan or design and its users. In their environment people see themselves reflected, or want to see themselves reflected. This is sometimes called reflexivity. People and agents play games with themselves, as well as with their environments, including other people. While playing games, people who adopt an ESS, increase their chances on evolutionary fitness. In this setting urban planners and architects can be seen as players, increasing their fitness by designing an environment for users, who in turn try to increase their fitness using that environment. In the preceding, one may replace people with agents (Minsky, 1988, p.17), generalizing the argument even further to include mindless processes, involving replicator molecules, viruses and cellular automata (Wolfram, 1983). The latter is especially appealing for incorporating the spatial dimension, which is essential to architecture and urban planning and design. Nowak and May (1992) were the first to play the Prisoners Dilemma game on the spatial grid of a cellular automaton. See Fig. 3.
77-78
Each cell (or pixel) with a particular strategy (indicated by the cell’s colour) plays a game against all its eight neighbours, accumulating payoff. This of course holds for the neighbours as well. When all payoffs are calculated, squares of nine cells are considered. The strategy of the cell with the highest payoff, will become the strategy of the middle cell in the next step. This process continues indefinitely yielding many beautiful patterns such as in Fig. 3.
The unit of a cellular automaton (or game) is the cell, elsewhere in this book identified with a pixel, which can be in a fixed number of states. When in two states the states are referred to as ON and OFF, usually coloured black and white and interpreted as cells being alive and dead. In an urban planning context the states could be houses (RED), parks (GREEN) and infrastructure (BLUE). On this small scale the rules one imposes govern the allowed state changes over one time step. A game in an urban setting would for example show changing patterns of red, green and blue. The power of these simple automatons are not to be underestimated, as Conway has shown that with the Game of Life rule set, one can construct a Universal Turing Machine. A Universal Turing Machine can mimic any computable function. With such functions one could construct an intelligent chess program which plays itself. All of this can follow from simple rules governing cells in state ON or OFF.
78
Which rules between cells apply in an architectural and planning context? The rules synthesising a design can sometimes literally represent processes. At other times they can merely be efficient algorithmic vessels to achieve real processes. The choice of rules also influences the predictability of the system. Take for example one dimensional cellular automaton rule 146. It is not predictable if one analyses the fine structure, but surprisingly it is predictable when one looks on a coarser level (Isreali and Goldenfeld, 2004), despite its complexity! Other rules can easily be derived from strict boundary conditions with regard to regulation, law, safety, collective functions, health, such as a rule prohibiting housing near large industrial facilities and heavy traffic. The search space that these rules allow accessibility to is still vast. One may research the remaining search space with help from a computer in general or by playing a game.
Since we are talking about games that are evolutionary, they exhibit all four phases of the evolutionary algorithm: mutation, variation, growth, selection. We would make a critical error to see this as merely change, in which no real innovation can occur. After all, are we humans not also changing patterns of atoms? The rules of the game are like the laws of physics. And the live cells are like the most elementary particles known to physics today: quarks and leptons. On this premise one can compare the evolutionary game grid with physical reality.
Concluding remarks
Do urban planners and architects need this new pair of glasses? The conclusion I draw is far from complete. But I have shown that it is possible for simple rules to yield innovative results. It may be hard to interpret the patterns from the game-cell level (= pixel level), as it would be equally hard to interpret a human being from the atomic level! Therefore the global level of a pixelized earth may already yield some innovative and interesting patterns, only observable when one has the mindset and tools to interpret them. In any case games and cellular automata help understand the levels of scale involved, and the way they are connected. It is in real-world multi-scaled and complex processes that contemporary architects and urban planners have to intervene. The gametheoretic point of view is a valuable contribution to a new pair of glasses.


Comentarios
Publicar un comentario